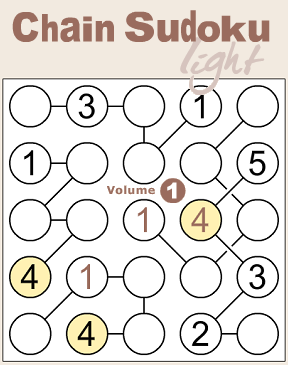When a work has an excellent premise, it's always interesting to find variations on the theme. Haydn knew it. Beethoven knew it. Braintonik knew it. Now Conceptis gets on board with another in their popular series of browser versions of pen-and-paper puzzles, and this time the spotlight is on likely the most popular remix of that ongoing phenom, Sudoku. Chain Sudoku Light Volume 1, presented with the same care as the others, is a twisty variant that's very, very fun.
Each puzzle consists of a square-grid of circles in which numbers are filled. Each individual circle is also connected in a chain which contains as many circles as the length/width of the grid. The object is to fill the grid with numbers (1 to 5, or 1 to 6, depending on the puzzle). so that each appears exactly once in each row, column and chain. Use the [mouse] to click on a circle and open a dial pad. Click on the dial pad for the desired number, or the X to clear the circle. Numbers can also be placed by typing. Pencilmarks to keep track of possibilities are also available. The toolbar at the top is for undoing, redoing, restarting, checking answers, showing solutions and saving.
Long-time readers may start to feel the praise is getting repetitive, but there's absolutely no doubt about it: Chain Sudoku light continues Conceptis' streak of excellent releases. The rules and general strategy should be quite familiar to any Sudoku-lover, but however small the differences in concept are, players will have to approach these new puzzles from a different mental direction. This makes for a perfect blend of fresh and familiar in the ol' noggin. Like many of Conceptis' "Volume 1" iteration, the difficulty is generous for beginners, and the included amount of puzzles (here, thirty) are over way too fast. Still, "Volume 1" implies a "Volume 2" is on the way, and, considering the quality, hopefully the authors won't be yanking our chains for too long.
Walkthrough Guide
(Please allow page to fully load for spoiler tags to be functional.)
Chain Sudoku For Novices: Tips and Strategies
The method I've found the most efficient is to pick one digit and look for rows, columns, and groups with only one position that digit could possibly fit. You could also look for circles where only one digit could possibly fit, but that's a little slower.
Because of the way the groups are shaped, a single circle outside of a group can share a row or column with a large fraction of that group. Even if you only have one instance of a given digit, that one instance can say a lot depending on where it is.
If a group takes up almost all of a row or column, the digits in the row or column but not in the group will be precisely the same as the digits vice-versa. A simple application of set theory will prove why. (Hint: if x+z = y+z, then...?)
Don't forget about pencil marks. If you know only two or three digits can belong in a circle, click the little square in the upper left of that circle and enter those numbers. They'll all show up without any one of them being finalized. This is a good way to remind yourself of what you've already figured out so you can eliminate possibilities elsewhere.
The fastest way to enter digits is to mouse over the circle (or the little square if entering pencil-marks) and press the appropriate number key on your keyboard (or the backspace key to erase). This way you don't have to deal with the little pop-up menu.
When you mouse over a circle, all the circles in the same group will be highlighted with a brown border. If you click on a digit you've already entered, all the identical digits will be highlighted in yellow, and you can use the arrow keys to cycle through the highlighted digits. Both of these very useful for checking at a glance what any digit's full influence is.
It can be very difficult to tell when you've made a mistake, so if you discover you've reached an impossibility it may be best to start over (the button with the two arrows). Or ragequit and go drown your frustration in a fresh cup of hot chocolate. Up to you.
Posted by: SonicLover
|
December 1, 2011 10:17 AM